
Dual cone and polar cone are closely related concepts in convex analysis, a branch of mathematics.


Dual cone and polar cone are closely related concepts in convex analysis, a branch of mathematics.
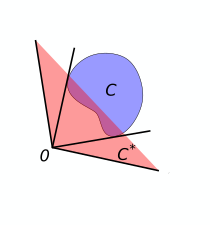
The dual cone C* of a subset C in a linear space X over the reals, e.g. Euclidean space Rn, with dual space X* is the set
where is the duality pairing between X and X*, i.e. .
C* is always a convex cone, even if C is neither convex nor a cone.
If X is a topological vector space over the real or complex numbers, then the dual cone of a subset C ⊆ X is the following set of continuous linear functionals on X:
which is the polar of the set -C.[1] No matter what C is, will be a convex cone. If C ⊆ {0} then .
Alternatively, many authors define the dual cone in the context of a real Hilbert space (such as Rn equipped with the Euclidean inner product) to be what is sometimes called the internal dual cone.
Using this latter definition for C*, we have that when C is a cone, the following properties hold:[2]
A cone C in a vector space X is said to be self-dual if X can be equipped with an inner product ⟨⋅,⋅⟩ such that the internal dual cone relative to this inner product is equal to C.[3] Those authors who define the dual cone as the internal dual cone in a real Hilbert space usually say that a cone is self-dual if it is equal to its internal dual. This is slightly different from the above definition, which permits a change of inner product. For instance, the above definition makes a cone in Rn with ellipsoidal base self-dual, because the inner product can be changed to make the base spherical, and a cone with spherical base in Rn is equal to its internal dual.
The nonnegative orthant of Rn and the space of all positive semidefinite matrices are self-dual, as are the cones with ellipsoidal base (often called "spherical cones", "Lorentz cones", or sometimes "ice-cream cones"). So are all cones in R3 whose base is the convex hull of a regular polygon with an odd number of vertices. A less regular example is the cone in R3 whose base is the "house": the convex hull of a square and a point outside the square forming an equilateral triangle (of the appropriate height) with one of the sides of the square.

For a set C in X, the polar cone of C is the set[4]
It can be seen that the polar cone is equal to the negative of the dual cone, i.e. Co = −C*.
For a closed convex cone C in X, the polar cone is equivalent to the polar set for C.[5]