 Involutional symmetry Cs, (*) [ ] = |
 Cyclic symmetry Cnv, (*nn) [n] = |
 Dihedral symmetry Dnh, (*n22) [n,2] = | |
| Polyhedral group, [n,3], (*n32) | |||
|---|---|---|---|
 Tetrahedral symmetry Td, (*332) [3,3] = |
 Octahedral symmetry Oh, (*432) [4,3] = |
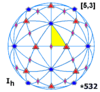 Icosahedral symmetry Ih, (*532) [5,3] = | |
In three dimensional geometry, there are four infinite series of point groups in three dimensions (n≥1) with n-fold rotational or reflectional symmetry about one axis (by an angle of 360°/n) that does not change the object.
They are the finite symmetry groups on a cone. For n = ∞ they correspond to four frieze groups. Schönflies notation is used. The terms horizontal (h) and vertical (v) imply the existence and direction of reflections with respect to a vertical axis of symmetry. Also shown are Coxeter notation in brackets, and, in parentheses, orbifold notation.


C2h, [2,2+] (2*) and C2v, [2], (*22) of order 4 are two of the three 3D symmetry group types with the Klein four-group as abstract group. C2v applies e.g. for a rectangular tile with its top side different from its bottom side.
In the limit these four groups represent Euclidean plane frieze groups as C∞, C∞h, C∞v, and S∞. Rotations become translations in the limit. Portions of the infinite plane can also be cut and connected into an infinite cylinder.
| Notations | Examples | ||||
|---|---|---|---|---|---|
| IUC | Orbifold | Coxeter | Schönflies* | Euclidean plane | Cylindrical (n=6) |
| p1 | ∞∞ | [∞]+ | C∞ | 
| |
| p1m1 | *∞∞ | [∞] | C∞v | 
| |
| p11m | ∞* | [∞+,2] | C∞h | 
| |
| p11g | ∞× | [∞+,2+] | S∞ | 
| |
| S2/Ci (1x): | C4v (*44): | C5v (*55): | |
|---|---|---|---|
 Parallelepiped |
 Square pyramid |
 Elongated square pyramid |
 Pentagonal pyramid |