Number equal to the sum of some of its divisors
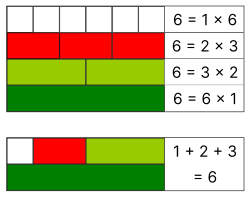
In number theory, a semiperfect number or pseudoperfect number is a natural number n that is equal to the sum of all or some of its proper divisors. A semiperfect number that is equal to the sum of all its proper divisors is a perfect number.
The first few semiperfect numbers are: 6, 12, 18, 20, 24, 28, 30, 36, 40, ... (sequence A005835 in the OEIS)
Primitive semiperfect numbers
A primitive semiperfect number (also called a primitive pseudoperfect number, irreducible semiperfect number or irreducible pseudoperfect number) is a semiperfect number that has no semiperfect proper divisor.[2]
The first few primitive semiperfect numbers are 6, 20, 28, 88, 104, 272, 304, 350, ... (sequence A006036 in the OEIS)
There are infinitely many such numbers. All numbers of the form 2mp, with p a prime between 2m and 2m+1, are primitive semiperfect, but this is not the only form: for example, 770.[1][2] There are infinitely many odd primitive semiperfect numbers, the smallest being 945, a result of Paul Erdős:[2] there are also infinitely many primitive semiperfect numbers that are not harmonic divisor numbers.[1]
Every semiperfect number is a multiple of a primitive semiperfect number.